Quantum engineered systems

Parametric systems

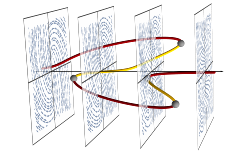
Parametric driving and its interplay with disorder and interactions in physical systems have led to numerous recent results, e.g., parametric resonance, synchronization, dynamical MBL, Floquet topological insulators, coherent destruction of tunnelling, and superfluid -insulator transitions in optical lattice systems. This contemporary research front opens up many opportunities as well as challenges. As initial steps in this direction, recently, we have revisited the simplest system of a harmonic oscillator subject to both parametric and external drives and were able to find novel sensing applications for nowadays technologies. Additionally, we have utilized parametric driving for proposing the generation of synthetic dimensions and gauges in atomic and photonic setups with prospects in bringing 4D topological physics to reality. Similarly, parametric drive is an inherent ingredient in realizing topological pumps, which we have demonstrated in both photonic and atomic setups. Last, we have predicted novel collective dynamic phases of parametrically driven light-matter systems.

As a result of our acquired experience and advanced methodology, we developed HarmonicBalance.jl -- a Julia package for solving nonlinear differential equations using the method of harmonic balance. Harmonic Balance methods efficiently approximate the dynamics of coupled resonator systems using a truncated Fourier expansion. The stationary states of the system are then obtained by solving a nonlinear system of equations that govern the amplitudes of the truncated ansatz. The package can assist numerous physics and engineering applications in out-of-equilibrium interacting systems.

• Floquet expansion by counting pump photons, K. Seibold, O. Ameye, and OZ [arXiv:2404.09704].
• Hamiltonian reconstruction via ringdown dynamics, V. Dumont, M. Bestler, L. Catalini, G. Margiani, OZ, A. Eichler, [arXiv:2403.00102].
• Limit cycles as stationary states of an extended Harmonic Balance ansatz, J. del Pino, J. Košata, and OZ, [arXiv:2308.06092].
• A biased Ising model using two coupled Kerr parametric oscillators with external force, P. Álvarez, D. Pittilini, F. Miserocchi, S. Raamamurthy, G. Margiani, O. Ameye, J. del Pino, OZ, and A. Eichler, [arXiv:2307.13676].
• Proliferation of unstable states and their impact on stochastic out-of-equilibrium dynamics, T. L. Heugel, R. Chitra, A. Eichler, and OZ, [arXiv:2307.13718].
• Khovanskii bases for semimixed systems of polynomial equations -- a case of approximating stationary nonlinear Newtonian dynamics, V. Borovik, P. Breiding, J. del Pino, M. Michałek, and OZ, Journal de Mathématiques Pures et Appliquées 182, 195 (2024) [arXiv:2306.07897].
• Deterministic and stochastic sampling of two coupled Kerr parametric oscillators, G. Margiani, J. del Pino, T. L. Heugel, N. E. Bousse, S. Guerrero, T. W. Kenny, OZ, D. Sabonis, and A. Eichler, Phys. Rev. Research 5, L012029 (2023) [arXiv:2210.14731].
• Fate of exceptional points in the presence of nonlinearities, A. Khedri, D. Horn, and OZ, [arXiv:2208.11205].
• The role of fluctuations in quantum and classical time crystals, T. L. Heugel, A. Eichler, R. Chitra, and OZ, SciPost Phys.Core 6, 053 (2023) [arXiv:2202.05577].
• Fixing the rotating-wave approximation for strongly-detuned quantum oscillators, J. Košata, A. Leuch, T. Kästli, and OZ, Phys. Rev. Research 4, 033177 (2022) [arXiv:2202.13172].
• HarmonicBalance.jl: A Julia suite for nonlinear dynamics using harmonic balance, J. Košata, J. del Pino, T. L. Heugel, and OZ SciPost Phys. Codebases 6 (2022) [arXiv:2202.00571].
• Extracting the lifetime of a synthetic two-level system, G. Margiani, S. Guerrero, T. L. Heugel, C. Marty, R. Pachlatko, T. Gisler, G. D. Vukasin, H.-K. Kwon, J. ML. Miller, N. E. Bousse, T. W. Kenny, OZ, D. Sabonis, and A. Eichler, Appl. Phys. Lett. 121, 164101 (2022) [arXiv:2112.03357].
• Strong parametric coupling between two ultra-coherent membrane modes, D. Hälg, T. Gisler, E. C. Langman, S. Misra, OZ, A. Schliesser, C. L. Degen, and A. Eichler, Phys. Rev. Lett. 128, 094301 (2022) [arXiv:2109.11943].
• Ising machines with strong bilinear coupling, T. L. Heugel, OZ, C. Marty, R. Chitra, and A. Eichler, Phys. Rev. Research 4, 013149 (2022) [arXiv:2103.02625].
• A distinctive class of dissipation-induced phase transitions and their universal characteristics, M. Soriente, T. L. Heugel, K. Arimitsu, R. Chitra, and OZ, Phys. Rev. Research 3, 023100 (2021) [arXiv:2101.12227].
• On the effect of linear feedback and parametric pumping on a resonator's frequency stability, Z. Mohammadi, T. L. Heugel, J. M. L. Miller, D. D. Shin, H.-K. Kwon, T. W. Kenny, R. Chitra, OZ, and L. G. Villanueva, New J. Phys. 22, 093049 (2020) [arXiv:2006.00650].
• Spin detection via parametric frequency conversion in a membrane resonator, J. Košata, OZ, C. L. Degen, R. Chitra, and A. Eichler, Phys. Rev. Applied 14, 014042 (2020) [arXiv:2003.04557].
• Dynamical phase transitions in driven-dissipative light-matter systems, M. Soriente, R. Chitra, and OZ, Phys. Rev. A 101, 023823 (2020) [arXiv:1909.09550].
• Rapid flipping of parametric phase states, M. Frimmer, T. L. Heugel, Z. Nosan, F. Tebbenjohanns, D. Hälg, A. Akin, C. L. Degen, L. Novotny, R. Chitra, OZ, and A. Eichler, Phys. Rev. Lett. 123, 254102 (2019) [arXiv:1905.11630].
• Classical many-body time crystals, T. L. Heugel, M. Oscity, A. Eichler, OZ, and R. Chitra, Phys. Rev. Lett. 123, 124301 (2019) [arXiv:1903.02311].
• A quantum transducer using a parametric driven-dissipative phase transition, T. L. Heugel, M. Biondi, OZ, and R. Chitra, Phys. Rev. Lett. 123, 173601 (2019) [arXiv:1901.03232].
• The 6D quantum Hall effect and 3D topological pumps, I. Petrides, H. M. Price, and OZ, Phys. Rev. B 98, 125431 (2018) [arXiv:1804.01871].
• A parametric symmetry breaking transducer, A. Eichler, T. L. Heugel, A. Leuch, C. L. Degen, R. Chitra, and OZ, Appl. Phys. Lett. 112, 233105 (2018) [arXiv:1803.10467].
• Exploring 4D Quantum Hall Physics with a 2D Topological Charge Pump, M. Lohse, C. Schweizer, H. M. Price, OZ, and I. Bloch, Nature 553, 55 (2018) [arXiv:1705.08371].
• Photonic topological pumping through the edges of a dynamical four-dimensional quantum Hall system, OZ, S. Huang, J. Guglielmon, M. Wang, K. Chen, Y. E. Kraus, and M. C. Rechtsman, Nature 553, 59 (2018) [arXiv:1705.08361].
• Parametric symmetry breaking in a nonlinear resonator, A. Leuch, L. Papariello, OZ, C. Degen, R. Chitra, and A. Eichler, Phys. Rev. Lett. 117, 214101 (2016) [arXiv:1608.08896].
• Ultrasensitive hysteretic force sensing with parametric nonlinear oscillators, L. Papariello, OZ, A. Eichler, and R. Chitra, Phys. Rev. E 94, 022201 (2016) [arXiv:1603.07774].
• Synthetic dimensions in integrated photonics: From optical isolation to 4D quantum Hall physics, T. Ozawa, H. M. Price, N. Goldman, OZ, and I. Carusotto, Phys. Rev. A 93, 043827 (2016) [arXiv:1510.03910].
• A Thouless quantum pump with ultracold bosonic atoms in an optical superlattice, M. Lohse, C. Schweizer, OZ, M. Aidelsburger, and I. Bloch, Nature Phys. 12, 350 (2016) [arXiv:1507.02225].
• Four-dimensional quantum Hall effect with ultracold atoms, H. M. Price, OZ, T. Ozawa, I. Carusotto, and N. Goldman, Phys. Rev. Lett. 115, 195303 (2015) (Editor's suggestion) [arXiv:1505.04387]. Featured in a Physics synopsis: The Quantum Hall Effect Leaves Flatland.
• Dynamical many-body phases of the parametrically driven, dissipative Dicke model, R. Chitra and OZ, Phys. Rev. A 92, 023815 (2015) [arXiv:1501.07098].
• Topological pumping over a photonic Fibonacci quasicrystal, M. Verbin, OZ, Y. Lahini, Y. E. Kraus, and Y. Silberberg, Phys. Rev. B 91, 064201 (2015) [arXiv:1403.7124].
• Topological states and adiabatic pumping in quasicrystals, Y. E. Kraus, Y. Lahini, Z. Ringel, M. Verbin, and OZ, Phys. Rev. Lett. 109, 106402 (2012) (Editor's suggestion) [arXiv:1109.5983]. It received a Physics viewpoint: Physics 5, 99 (2012) and was chosen as highlighted research in Science 338, 444 (2012) and Nature Physics 8, 702 (2012). It has also featured in physicsworld and has an invited article in 2Physics.